[LeetCode] 329. Longest Increasing Path in a Matrix

轉自LeetCode

Given an m x n integers matrix, return the length of the longest increasing path in matrix.

From each cell, you can either move in four directions: left, right, up, or down. You may not move diagonally or move outside the boundary (i.e., wrap-around is not allowed).

 

Example 1:

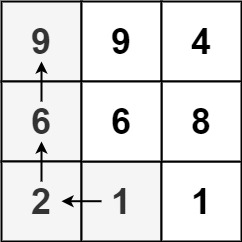
Input: matrix = [[9,9,4],[6,6,8],[2,1,1]]
Output: 4
Explanation: The longest increasing path is [1, 2, 6, 9].

Example 2:

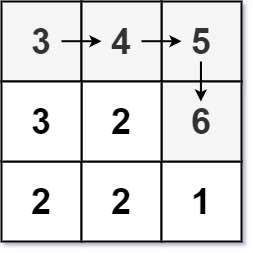
Input: matrix = [[3,4,5],[3,2,6],[2,2,1]]
Output: 4
Explanation: The longest increasing path is [3, 4, 5, 6]. Moving diagonally is not allowed.

Example 3:

Input: matrix = [[1]]
Output: 1

 

Constraints:

• m == matrix.length
• n == matrix[i].length
• 1 <= m, n <= 200
• 0 <= matrix[i][j] <= 231 - 1

Solution

這題直覺想到用 DFS 來做，但是會TLE，所以要加速一下

這次要使用一個 DP 來加速，dp[i][j] 表示從 matrix[i][j] 出發的最大長度

所以在 DFS 的過程當中，如果 dp[i][j] 值已經不是初始的 0，那就直接回傳該值就好

kotlin