[LeetCode] 375. Guess Number Higher or Lower II

轉自LeetCode

We are playing the Guessing Game. The game will work as follows:

1. I pick a number between 1 and n.
2. You guess a number.
3. If you guess the right number, you win the game.
4. If you guess the wrong number, then I will tell you whether the number I picked is higher or lower, and you will continue guessing.
5. Every time you guess a wrong number x, you will pay x dollars. If you run out of money, you lose the game.

Given a particular n, return the minimum amount of money you need to guarantee a win regardless of what number I pick.

 

Example 1:

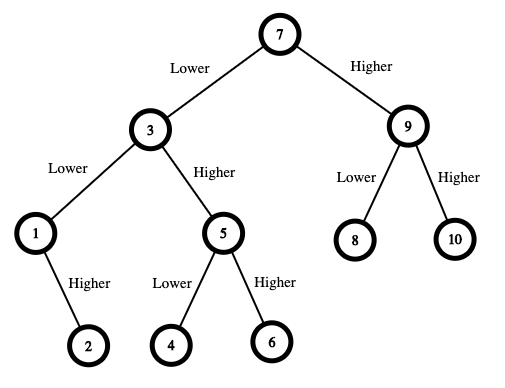
Input: n = 10
Output: 16
Explanation: The winning strategy is as follows:
- The range is [1,10]. Guess 7.
    - If this is my number, your total is $0. Otherwise, you pay $7.
    - If my number is higher, the range is [8,10]. Guess 9.
        - If this is my number, your total is $7. Otherwise, you pay $9.
        - If my number is higher, it must be 10. Guess 10. Your total is $7 + $9 = $16.
        - If my number is lower, it must be 8. Guess 8. Your total is $7 + $9 = $16.
    - If my number is lower, the range is [1,6]. Guess 3.
        - If this is my number, your total is $7. Otherwise, you pay $3.
        - If my number is higher, the range is [4,6]. Guess 5.
            - If this is my number, your total is $7 + $3 = $10. Otherwise, you pay $5.
            - If my number is higher, it must be 6. Guess 6. Your total is $7 + $3 + $5 = $15.
            - If my number is lower, it must be 4. Guess 4. Your total is $7 + $3 + $5 = $15.
        - If my number is lower, the range is [1,2]. Guess 1.
            - If this is my number, your total is $7 + $3 = $10. Otherwise, you pay $1.
            - If my number is higher, it must be 2. Guess 2. Your total is $7 + $3 + $1 = $11.
The worst case in all these scenarios is that you pay $16. Hence, you only need $16 to guarantee a win.

Example 2:

Input: n = 1
Output: 0
Explanation: There is only one possible number, so you can guess 1 and not have to pay anything.

Example 3:

Input: n = 2
Output: 1
Explanation: There are two possible numbers, 1 and 2.
- Guess 1.
    - If this is my number, your total is $0. Otherwise, you pay $1.
    - If my number is higher, it must be 2. Guess 2. Your total is $1.
The worst case is that you pay $1.

 

Constraints:

• 1 <= n <= 200

Solution

374. Guess Number Higher or Lower 的衍生題

想法和 329. Longest Increasing Path in a Matrix 類似

需要用到 DFS，並搭配 DP 來加速

dp[i][j] 表示從數字 i 到 j 的必贏的 cost

每次 recursion，把數列分成左半和右半，找出如果猜錯當下數字時，最大的 cost 是多少

然後從這些最大 cost 中，找出最小的 cost

Kotlin(參考解法)

	class Solution {
	fun getMoneyAmount(n: Int): Int {
	val dp = Array(n+1) { IntArray(n+1) {0}}
	return dfs(1, n, dp)
	}
	fun dfs(start: Int, end: Int, dp: Array<IntArray>): Int {
	return when {
	start >= end -> 0
	dp[start][end] > 0 -> dp[start][end]
	else -> {
	var result = Int.MAX_VALUE
	for(i in start..end) {
	val cost = i + Math.max(dfs(start, i-1, dp), dfs(i+1, end, dp))
	result = Math.min(result, cost)
	}
	dp[start][end] = result
	result
	}
	}
	}
	}

view raw guess_number_higher_or_lower_II.kt hosted with ❤ by GitHub